Gaspard Monge was a French mathematician of the 18th and 19th century, a man of many parts who was a friend of Napoleon and took part in the latter's expedition to Egypt. He gave his name to a theorem in geometry which is easy to see in this illustration from Wikipedia (image by Jason Quinn).
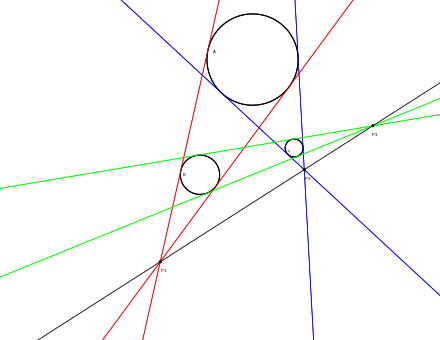
Although it is usually presented as as theorem about circles and tangents it seems more general than that. Here is my drawing of an analogue for equilateral triangles, produced using GeoGebra.

For any pair of triangles, the lines drawn through pairs of corresponding points meet at a point, and for any set of three triangles taking the triangles in pairs produces three such points that all lie on the same line.
I don't think equilateral triangles are special, as far as I can see we could choose any shape.
Monge's theorem can also be generalised to three dimensions (four spheres and six cones, one for each pair of spheres, all of whose vertices lie on a common plane), and indeed to higher dimensions, although it obviously gets harder to visualise.