This is an addendum to some aide-mémoire notes I am making on Relativity. Perhaps somebody may find it useful. Nowadays aide-mémoire has become more and more of a requirement for me.
In Special Relativity (SR), gamma (Greek lower case), aka the Lorentz Factor, signifies the multiplier required to obtain a dilated (increased) time interval between two co-located events in inertial frame A as observed from another inertial frame B where A and B are in relative motion. Time dilation increases with relative velocity so gamma can be written as a function . Gamma is ubiquitous in SR calculations.
Observers in both frames will measure the same spacetime separation between events occurring in either frame but, to allow a simple time-only translation using just , the events in A must be co-located: – in the same spatial location in the frame, that is.
Time effects and most other outcomes of SR are symmetrical. If A sees B’s clock running slow then B will see A’s clock slow. This must be so to maintain the relativity principle: the motion of any entity can only be defined in relation to another entity and consequently absolute motion or rest have no meaning. With all motion being relative, observations made from one frame must mirror those made from the other.Similarly, light (electromagnetic radiation) is not subject to Galilean velocity addition and has the same absolute speed for all observers moving or otherwise. This is a requirement if electromagnetic phenomena are to show the same results in all locations as indeed they do. Both these requirements need additional examination elsewhere.
The derivation
Most derivations, apart from Einstein (1905 p7), feature light clocks and Einstein does appear to mention the elongated light path seen in a moving frame:
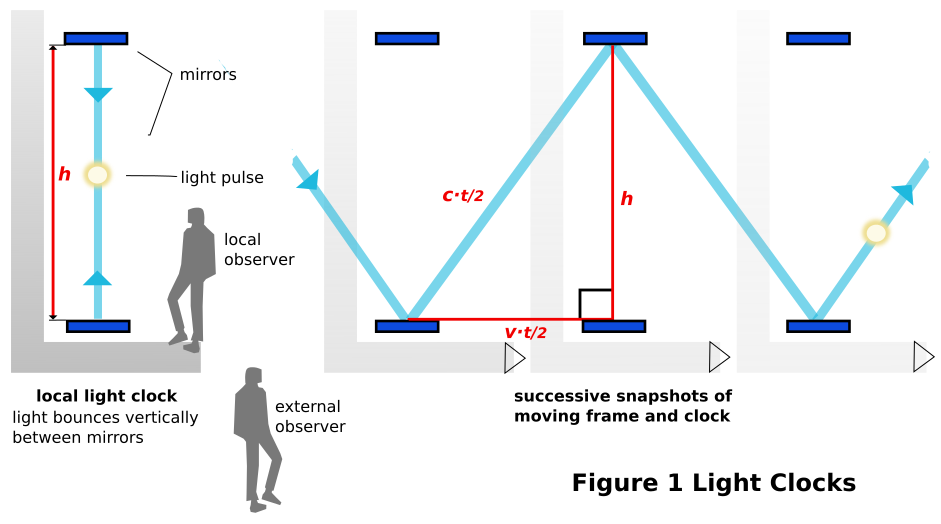In fact
the mirrors, shown vertically spaced, can have any orientation at 90º
to the direction of travel.
The norm is to consider a full clock cycle where a light pulse leaving the bottom mirror is reflected off the top mirror and returns. The ratio between the length of half a cycle in the stationary clock and half in the moving clock is obviously the same but a possible justification for the full-cycle approach is to emphasize that the two events, leaving and returning, must be co-located. Following this option:
If the
time taken for a full cycle of the stationary clock is , the
distance the light pulse travels at velocity between the mirrors is:
Similarly, in the moving clock if is the time taken for a full cycle, the increased distance the light travels along a single diagonal is . The distance the clock has moved during half a cycle is where is the relative velocity of the moving frame. The spacing of the mirrors remains unchanged. Applying Pythagoras’ theorem to the right triangle in Figure 1 we can equate light travel distance to and :
Combining equations (1) and (2)
expanding the brackets
then multiplying both sides by 4 and dividing by
rearranging gives
multiplying both sides by -1
take out
square root both sides
or may be preferred.
From this, the period of one (or n) clock cycles of the local clock is equal to the period of the same number of clock cycles of the moving clock multiplied by . The longer a clock cycle the slower the clock will run so dilated intervals for this clock as seen by an external observer will involve the reciprocal
where and are the intervals between events seen in their respective frames.
Examples
gives
intervals, as seen by an observer in frame A, between
co-located events in B. If a spaceship B has a relative
velocity of half the speed of light relative to space-station A then
Say that after every minute that passes by shiptime B transmits a time signal – these transmissions constitute co-located events in B. Standing off at right angles to B’s course to minimize any Doppler effect, space-station A receives signals at 1.1547 minute intervals. So, for every 1.1547 minutes of time that passes in A only 1 minute has elapsed in B.
To obtain the reduced time period experienced in moving frame B we need the reciprocal of gamma:
From A’s perspective it will take B a year to travel half a lightyear but for B the time elapsed is 1/1.1547 = 0.8660 years.
Acknowledgement
The above derivation is based on Gray (2022) expanded and annotated with basic examples. Any misunderstandings and errors are without doubt my own.
References
Einstein, A. (1905) On the Electrodynamics of Moving Bodies. (trans. Dr. Anna Beck, and consultant Professor Peter Havas), Princeton Digital Einstein Papers [online] https://dn790004.ca.archive.org/0/items/einstein-1905-relativity/Einstein_1905_relativity.pdf
Gray, N. (2022) A Student’s Guide to Special Relativity, Cambridge University Press