Wiktionary defines an antiproverb as: " A humorous adaptation of one or more existing proverbs."

There are many forms but a common one starts with one proverb, then switches in midstream to another. For example, the daftly incongruous

"Every dog has a silver lining"

Or the sardonic

"No news is the mother of invention."

Here are more examples from [1]

Don’t count your chickens in midstream
You can lead a horse to water, but you can’t have it both ways.
Too many cooks are better than one.
An apple a day is worth two in the bush.

The word antiproverb was coined by Wolfgang Mieder and there is quite a literature about antiproverbs ^[2][3]. People who study proverbs are paremiologists, a word new to me but paremiology is in the OED and attested from 1861, derived from Latin paroemia, Greek παροιμία.

I thought I would try to generate some antiproverbs of my own, so I got a list of just under 1,000 proverbs and generated many random pairs, looking for good combinations. It turned out harder than I was expecting, but here are some that I feel show definite promise

An apple a day is better than no bread.
Don't count your chickens while the sun shines.
Many a true word is sauce for the gander.
Don’t change horses till the fat lady sings.
It’s an ill wind that never boils.
It’s easy to be wise after a free lunch.

[1]  https://wordsbybob.wordpress.com/2014/01/20/antiproverbs-say-what/

[2] https://www.degruyterbrill.com/document/doi/10.2478/9783110410167.15/html

[3] https://www.researchgate.net/publication/370487535_ENGLISH_ANTI-PROVERBS_AS_STYLISTIC_DEVICES

More than 2,000 years ago Euclid proved that in any triangle the three lines bisecting the angles of a triangle meet at a point which is the centre of the circle that touches the triangle's sides.

He further proved that the three lines that bisect the triangle's sides at right angles is the centre of a circle passing through the triangles three corners.

These circles, called the incircle (centre incentre) and circumcircle (centre circumcentre) still studied in schools today, for example here is quite a nice animation showing the construction of the circumcircle, from a GCSE revision site.

And if you are interested in Euclid's original proofs here you can see the relevant pages from the oldest know complete copy of Euclid's Elements, with a transcription into a readable form and an English translation. You want Book IV, Elem. 4.4 and 4.5. This website is an astonishing work of scholarship.

All that was just the preamble. Here is a neat fact I stumbled across about a week ago, when I was just doodling triangles. It's nice because it connects the angle bisectors and the perpendicular bisectors.

In a triangle the line bisecting an angle meets the perpendicular bisector of the opposite side at a point (M in the diagram below) that lies on the circumcircle. 

This was new to me but I thought there ought to be quite a simple and accessible proof. But after a bit of head scratching, I couldn't see one, so I thought it must be a standard result, and looked it up. I did find a few proofs, but they were all more complicated than I was hoping (and at least one was wrong). The problem is discussed on Mathematics Stack Exchange but I still didn't find the "obvious" proof I was looking for.

After days of head-scratching I finally had my eureka moment! The proof I was seeking uses the following fact.

In a given circle, any two chords with the same length subtend (i.e.make) equal angles on the circumference. Here's an example:

Now it's easy. Add some chords.

I claim that the line BM that joins B and the point M where the perpendicular bisector of AC meets the circumcircle is the line bisecting angle ABC.

Proof: Any point on the perpendicular bisector of AC is equidistant from A and C. So AM and MC are equal chords, and the angles ABM and MBC they subtend are equal, in other words BM bisects angle ABC.

I saw this problem on the "Mind Your Decisions" YouTube channel and here are my two solutions, I haven't watched the video/

The Problem

In my sketch we have a rectangle of unknown dimensions, a quarter-circle inscribed in the rectangle, a semicircle positioned as shown with its centre on bottom of the rectangle, and a line of length 5 drawn from the corner of the rectangle and tangent to the semicircle. The challenge is to find the area of the rectangle.

At first sight this is impossible, we only have one distance so how can we find the area? 

First Solution

When a problem involves a tangent to a circle or part of one, it almost always uses the fact the a tangent makes a 90 degree angle with the radius at the point of contact; and when a problem involves a right-angled triangle and we are interested in distances, that points to Pythagoras' Theorem.

So here's the diagram again: I have labelled some key points, called the radii of the quarter and semicircle r₁ and r₂ respectively, drawn in the radius to point of contact D, and marked the right angle.

Now we see we have a right-angles triangle with hypotenuse r₁ + r₂ and its other sides 5 and r₂. We can apply Pythagoras and then use some algebra on the resulting equation as follows.

But r₁ and r₁ + r₂ are precisely the height and length of the rectangle and their product is the area of the rectangle, which must therefore be 25. r₁ and r₂ can take different values as long as the satisfy the relationship we ended up with above and the area must always be 25.

Second solution

We are not told the values of r₁ and r₂, so it must not matter as such and we are free to choose them as we like as long as our choice is compatible with the given geometry.

Very well: let's set r₂ = 0. Now the semicircle collapses to a point at B, the rectangle becomes a square, and the tangent degenerates into the line AB, r₂ becomes 5 and the area is (r₂)² = 25.

It's a bit of a mystery.

In most of the languages of Europe the word for dog comes from an ancient PIE root which was something like kwon- and which appears in e.g. Latin canis, Ancient Greek kyon and Welsh corgi. In Germanic languages the k sound became h, so we get Modern German Hund and Old English hund (another example of this consonant shift is Greek kardia versus English heart, German Hertz).

For today 'hound' is reserved for dogs (or people) that hunt or track something bloodhound, newshound, or breeds of hunting dogs wolfhound, or used metaphorically, or perhaps in a jokey way. At some point in late Old English a word docga (possibly referring to a spacial breed of strong or powerful animal) emerged from completely unknown origins. During the Middle English period dog displaced hound as the standard word for members of the genus Canis and the meaning of hound narrowed to the more restricted sense it is used in today.

To me hound has more portentous feel than dog: "The Dog of the Baskervilles" wouldn't really worked.

A palindrome is of course a word (e.g. tattarrattat, a knock at the door) or phrase (e.g. Norma is as selfless as I am Ron, my favourite) that reads the same forwards and backwards,.

But have you heard of the anadrome? An anadrome is a word the taken backwards gives a different, but perfectly good, word. The longest example in English is, as far as anyone knows, the 8-letter stressed and desserts.

I wrote a short program that searched a public-domain word list and found several 7-letter anadromes:

dessert
reviver
reifier
stinker
stellas
deifier
deified
deliver
reviled
rewarder
halalah
reified
sallets
reknits
stressed
sememes
redrawer
tressed

I rather like stinker and reknits.

If we consider shorter words there are many more anadromes: I found 397 of 2 or more letters in a list of 113809 words.

There are also some that have been made up: the unit of electrical resistance is the ohm and as far back as 1888 someone coined mho as a unit for the reciprocal of resistance, conductance. It's probably not official but I think it's quite common, I was certainly familiar with it. Another nice example I found on Wikipedia is tink, which means unknit (geddit?). This goes rather nicely with stinker and reknits, I feel.

Suppose we have a long piece of wood with a uniform cross-section which is an irregular triangle (Fig. 1), so a straight cut at right angles to the length of the timber results in a triangle all of whose sides are different.

Show that it is always possible to make an oblique cut at an angle in such a way that the section obtained is an equilateral triangle (Fig. 2).

This comes from the Turkish Maths Olympiad 2000 and I read about it here, p. 141. 

An outline proof appears in the comments.

This plant, which growing profusely in my street, is Red Valerian, Valeriana Rubra (or Centranthus ruber). Originally from the Mediterranean, it has been introduced into many other parts of the globe and become widely naturalised.

The genus Valeriana was named by Linnaeus, after the Roman Emperor Valerian. Valerian means "Worthy or "Strong" and descends from the same root as "Value", "Valid", Valour" etc. Linnaeus chose this name because another Valerian species, Valeriana officinalis has been used since the time of Hippocrates as a herbal remedy with a range of uses, so I suppose he felt it deserved the description of "Worthy".

Two interesting facts about V. officinalis.

1. It's attractive to cats, like catnip.

2. An old Swedish country superstition was that (it is so fascinating I shall quote it in full)

'… a bridegroom stands in dread of the envy of the Elves, to counteract which it has long been a custom to lay in the clothes on the wedding day certain strong-smelling plants, as garlic or valerian. Near gates and in crossways there is supposed to be the greatest danger. If any one asks a bridegroom the reason of these precautions, he will answer : " On account of envy." And there is no one so miserable whose bride will not think herself envied on her wedding day, if by no others, at least by the Elves.'

The elves would spirit the groom away

' The bride sits ready in her bridal bower, in anxious ex pectation and surrounded by her bridesmaids. The bride groom saddles his grey steed, and clad in knightly attire, with his hawk perched proudly on his shoulder, he rides forth from his mother s hall, to fetch home his bride. But in the wood where he is wont to hunt with hawk and hound, an elfin maiden has noticed the comely youth, and is now on the watch for an opportunity, though for ever so short a time, to clasp him to her breast in the flowery grove; or, at least, to the sweet tones of their stringed instruments, lightly to float along with him, hand in hand, on the verdant field. As he draws near to the elf-mount, or is about to ride through the gateway of the castle, his ears are ravished with most wondrous music, and from among the fairest maidens that he there sees dancing in a ring, the Elf-king s daughter herself steps forth fairer than them all, as it is said in the lay :

The damsel held forth her snow-white hand : " Come join in the merry dance with me."

If the knight allows himself to be charmed, and touches the fascinating hand, he is conducted to Elfland, where in halls indescribably beautiful, and gardens such as he had never beheld, he wanders about, on his Elf-bride s arm, amid lilies and roses. If at length the remembrance of his mourning betrothed enters his mind, and the Elves, who do not deliberately desire evil to mankind, are moved'

From 

Northern mythology : comprising the principal popular traditions and superstitions of Scandinavia, North Germany, and The Netherlands, volume 2  

SCANDINAVIAN POPULAR TRADITIONS AND SUPERSTITIONS (1851), VOL: 2, OF ELVES, p. 62 https://www.artandpopularculture.com/Northern_mythology_:_comprising_the_principal_popular_traditions_and_superstitions_of_Scandinavia,_North_Germany,_and_The_Netherlands,_volume_2

For some reason I wondered about the origins of "go" and when I looked it up found a discussion of why the past tense of "go" is "went" rather than "goed". It is rather strange.

In Old English "go" was gan, the ancestor of the modern word, and it already had an irregular plural eode, a word of unknown origin.

At that time "went" was the past of wendan, connected with modern wind (as in turn). "Wend" has survived into Modern English as "wend", as in wend ones way. Nowadays the word has narrowed its meaning to imply travelling in a circuitous and roundabout way and has a rather archaic ring, but originally seems to have meant wind in the general sense.

"Wend" had a past tense "went" (like spend -> spent; send -> sent; lend -> lent; rend (meaning rip) -> rent and probably others) and for some reason is Early Middle English this started to be used in place of eode, which was eventually replaced, and disappeared from the language.

But why was "goed" not adopted? One possibility could be to avoid confusion with "good" which would at that point have had a vowel sound like that in "oak" and a 'g' like that in "go". Just a theory, I don't really have a grasp on these sound changes.

As a wordplay enthusiast I've often mused over what words can be formed by combining the symbols of the chemical elements.

For example, Actinium-Actinium-Iodine-Arsenic would give "acacias" and Barium-Oxygen-Barium-Boron-Sulphur would give "Baobabs".

A while ago I did try to come up with some  examples by hand but only found a few, so I decided to write a little program in Python.(see below[*]). For simplicity I just used lowercase letters e.g. "h", "he", "li" etc. but putting back the capitals would not be too hard. I also excluded the artificial transuranic elements (26 found to date) but again adding them would be easy enough. My reason for omitting them is that their name and symbols are a bit less familiar.

Also note it uses a "greedy" algorithm and if the first two letters is a valid symbol it chooses that first, so it can't handle "those", because it finds "th" (Thorium), then "os" (Osmium), and now the "e' is orphaned. To overcome this I'd need to add some backtracking capability so the program gets a bit more complex.

I analysed an open-source word list of 113,810 words and found 13,435 - let's call them "elementary" - words, about 12%. We'd expect the chances of a word being elementary to fall off as words gets longer, because only 92 one- or two-letter combinations of letters are symbols of chemical elements but the total number of possible one- or two-letter combination is 702, so only about 1 in 8 correspond to elements.

Nonetheless I found some surprisingly examples, with the champion being the 16-letter "counteraccusations". Here are some other long ones.

acacias
acarpous
accepters
accessions
accountancy
accurateness
accuratenesses
articulatenesses
counteraccusation

"counteraccusations" represents Cobalt Uranium Nitrogen Tellurium Radium Carbon Copper (Cu) Samarium Titanium Oxygen Nitrogen Sulphur; 11 distinct elements which I imagine is a record.

What about whole sentences? It's not easy to make up natural-sounding ones, but here is my attempt at one from a scientific setting. I've added proper punctuation to make it more realistic.

"Pop both new boxes in lab one Gabby, ta."

[*] Program follows: list of symbols, then function to test words  then sample function calls.

elements = [
    "h", "he", "li", "be", "b", "c", "n", "o", "f", "ne",
    "na", "mg", "al", "si", "p", "s", "cl", "ar", "k", "ca",
    "sc", "ti", "v", "cr", "mn", "fe", "co", "ni", "cu", "zn",
    "ga", "ge", "as", "se", "br", "kr", "rb", "sr", "y", "zr",
    "nb", "mo", "tc", "ru", "rh", "pd", "ag", "cd", "in", "sn",
    "sb", "te", "i", "xe", "cs", "ba", "la", "ce", "pr", "nd",
    "pm", "sm", "eu", "gd", "tb", "dy", "ho", "er", "tm", "yb",
    "lu", "hf", "ta", "w", "re", "os", "ir", "pt", "au", "hg",
    "tl", "pb", "bi", "po", "at", "rn", "fr", "ra", "ac", "th",
    "pa", "u"
]

def parse(word):

    result = []

    while len(word) > 0:

        token1 = word[0:1]
        token2 = word[0:2]

        # Look for two-letter symbol first
        if token2 in elements:
            result.append(token2)
            word = word[2:]

        # If not check for 1--letter symbol 
        elif token1 in elements:
            result.append(token1)
            word = word[1:]

        else:
            return 'fail'
        
    return result

print(parse('cream'))
print(parse('scone'))

Equilateral triangles ABC and CDE share a common vertex at C. Prove that AD and BE are equal in length.

Solution tomorrow.

Every now and again someone comes up to me in a pub or a hotel or a classroom and sketches this little picture.

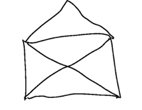"Can you draw this", they say, "without going over the same bit twice or lifting the pen off the paper?"

"I can", I say, and then I try to explain how I can be so confident without even picking up the pen. Here's how it goes.

First identify each point where two of more lines meet.

Now count how many lines meet at each point.

For it to be possible to trace the figure it's necessary that either:

• All the numbers are even, or;
• Two and only are odd (as is the case here) and you start from one of these and end at the other. 

This is because every time you pass through a point you add 2 to the count. If you start and finish at the same point then an extra 1 is added when start and another 1 again when you finish, so all the counts are even. Otherwise if we start and finish at different points, both get an extra 1, so their final count will be odd but all the other counts even.

Providing one of these holds it can be proved (but I'm not going do so here, it's not that hard but a bit too long) that the figure can be traced. And here's a solution (not the only one). The points with an odd count are highlighted.

These ideas were first articulated by Leonhard Euler in 1736. A problem that must have been going the rounds at the time concerned the seven bridges of Königsberg (in Prussia then; now Kaliningrad in Russia). The puzzle was: is it possible to find a tour that crossed each bridge exactly once.

Here are the bridges as shown in Euler's original paper, which you can find in translation here.

Out of interest I looked for the bridges on Google Earth and as you can see only five are still there; b and d were presumably destroyed in WW II. I hope I have identified the other five correctly.

Now if we take A, B , C and D to be points, and the original seven bridges a - g as lines connecting the points, we get this figure, and the problem of the Königsberg bridges becomes one of tracing the figure without crossing any bridge twice or taking the pen off the paper.

All four of A - D have an odd number of lines meeting at them, so from the discussion given earlier the puzzle of the seven bridges has no solution, although with only the five bridges that have survived it does become possible to make a tour of the desired kind, starting at A and ending at D, or vice versa.

Euler called his paper, which has since become very famous, "SOLUTION OF A PROBLEM IN THE GEOMETRY OF POSITION" and it gave rise to the branch of mathematics now called graph theory: nothing to do with x and y-axes, or plotting lines and parabolas etc., but concerned with the properties of arrangements consisting of points (vertices) connected by lines (edges). As well as being of great intrinsic interest and beauty, graph theory is a vital tool in the design of the algorithms and data structures behind the computer programs that play such an important role in modern life.

My friend heard a song by Duffy and when she sang

what he heard was

"Mercy" makes better sense, people don't often beg for birdseed, but that's not necessarily how Mondegreens work.  In The Language Instinct Steve Pinker mentions Mondegreens and notes that the mishearing is often fits the context less well than the original word and this seems be an example of that.

Yet perhaps a singer begging for birdseed does have some kind of underlying logic, when you think about it.

"AI Overview" in Chrome says a polyhedron is:

"a three-dimensional geometric shape with flat polygonal faces, straight edges, and sharp corners or vertices"

which is not a bad definition in my view. Some of these polyhedra are familiar to us; for example here is a back-of-an-envelope sketch of some with 4, 5 and 6 faces.

Note that we are not concerned here with the particular angles or side lengths, just with the topology: how many faces and vertices there are, how many sides each face has, and what faces fit with what other faces around each vertex. 

The solid with 4 faces - a tetrahedron aka triangular pyramid - is the only possibility for 4 faces, and for 5 faces there are exactly two polyhedra - the pyramid with a quadrilateral base and the triangular prism.

The two hexahedra shown are not the only ones however. There are 7 altogether; can you find some or all of the remaining ones? Solution in the Comments.

As the number of faces grows the number of possible polyhedra climbs exponentially [1].

Numbers up 10 faces are exact, but those from 11 on are only estimated.

Amazingly Steven Dutch has enumerated and classified all 2606 enneahedra, finishing on 2 November 2016 [2].

Here's a quick question to finish - what is a regular hexahedron better known as?

References

[1] https://oeis.org/search?q=A000944&language=english&go=Search

[2] https://stevedutch.net/symmetry/polynum0.htm