No round holes involved, the Square Peg Problem is a deceptively simple mathematical problem, first posed over a hundred years ago. It asks whether every closed curve has an inscribed square, that is, a square with all four of its vertices on the curve, as in the example below.
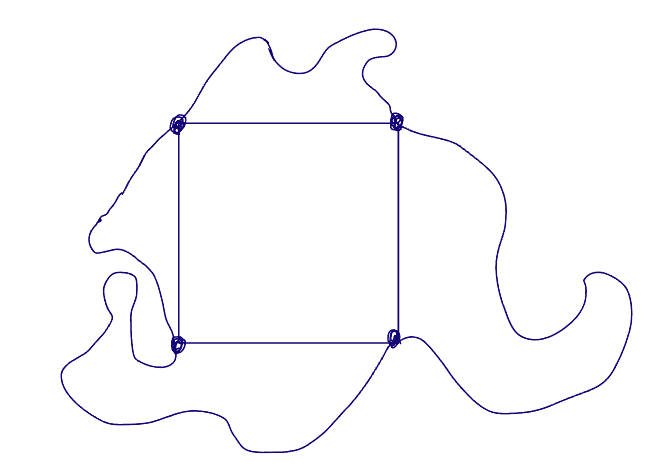
I've been fascinated by this problem for nearly 50 years. It's easy to state but hard to solve and although solutions have been found for many particular categories of curve, a completely solution is still lacking and it remains an active research topic. There is a good summary of the history and current state of the problem here:
https://diposit.ub.edu/dspace/bitstream/2445/151918/2/151918.pdfRecently I remembered a problem someone showed me many years ago. Given an acute triangle can you always inscribe a square in it? The answer is yes and with the right insight it’s not hard to see why.
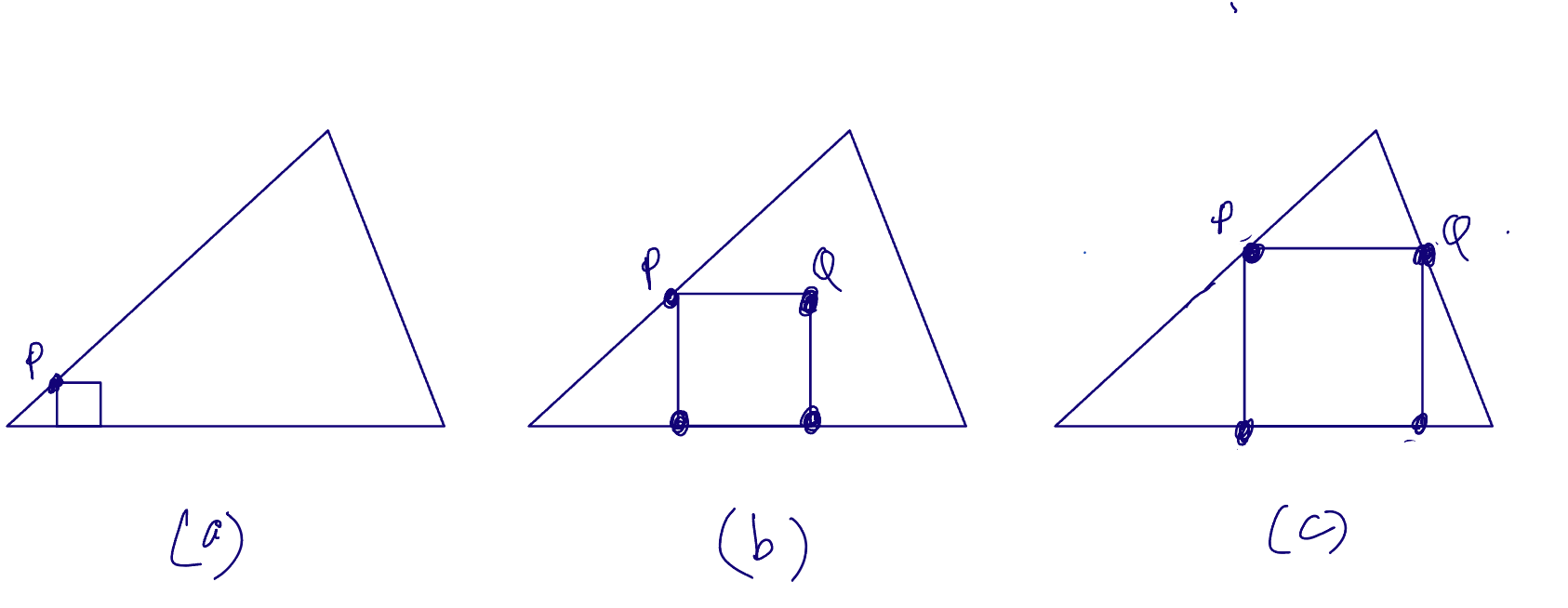
The idea is to begin at (a), with a small square that has three vertices on the triangle, the point P and two others on the base of the triangle. In (b) we move P along the side it lies on, while maintaining the same square configuration, and at some stage the point Q must meet the third side of the triangle (c), and we have the desired inscribed square. Rather neat.
It occurred to me that this was a special case of the square peg problem, so I went off and caught up on the latest research, and the summary I referred to above reproduces a number of proofs for different classes of curve. Most of them are quite difficult, some very, but I found one that looked as though it ought to be easy, although I still struggled with it a bit. I wanted to write something about the square peg problem and give a simple and intuitive proof for at least one case, but this one seemed to involve too much maths to be generally accessible.
After a couple of days pondering this, I woke up suddenly in the early hours of the morning with a eureka moment. The proof I'd being looking at is really just the same as the triangle problem above. There is really nothing special about the triangle, it could be any path that starts at a base level, has some ups and downs, and eventually end up at the base level again. It might be a section through a hill for example, like this:
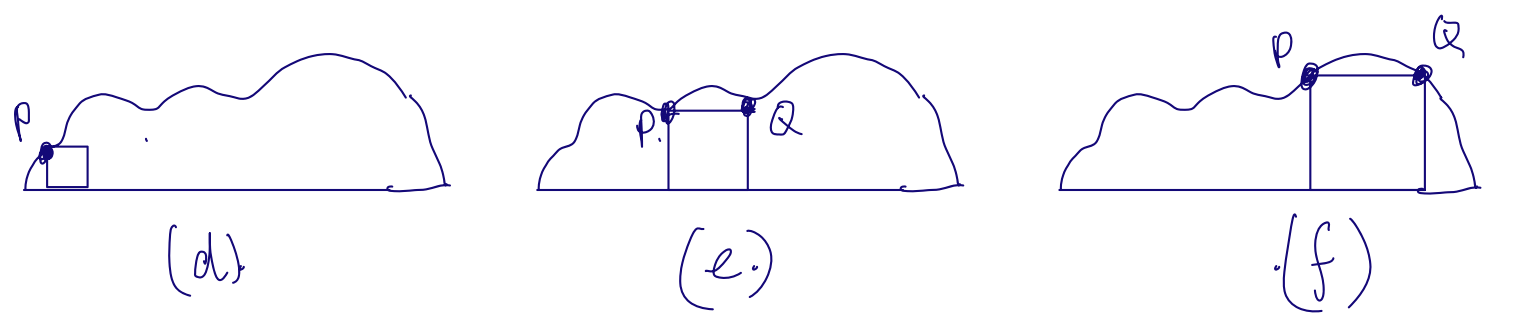
As before we draw squares with two vertices at base level, and a third vertex P which lies on the hillside. We start with a small square, as shown in (d) and move P along the surface of the hill as in (e). In (e) point Q just misses being on the surface, but we carry on and eventually there must come a time, as shown in (f), when Q meets the surface, and we have an inscribed square. Of course we would need to tighten this argument up a bit before it became a rigorous proof, but it's basically correct and quite easy to follow.
Staying with idea of the hill section, the inscribed square means there must necessarily be two points on the hillside with the same elevation whose distance apart equals that elevation.